Modeling the fall of an object with air resistance
Annemarie Branks
Professor Wolf
Objective: Determine the relationship between air resistance and speed. Model the fall of an object including air resistance.
Procedure:
1. We want to be able to drop an object that would be subjective to air resistance but still be able to drop straight down without moving left and right like a feather would. Coffee filters worked out great for this experiment. We need to find the object's terminal velocity so we have to drop it from a high enough location so that can happen.
2. For each trial we dropped an increasing number of coffee filters from a high balcony while recording a video of the experiment on LoggerPro. The person who dropped the coffee filters also held a meter stick so that we could tell LoggerPro how long a meter is in the image. We stopped the experiment after dropping five coffee filters together.
3. In LoggerPro we made a position graph for each trial and highlighted the section with a relatively straight slope and made a linear fit. This slope is the experimental velocity for a given amount of coffee filters dropped. Below is an example of the experimental velocity for four coffee filters dropped.
4. From the overall look of the position graph we are assuming that the Force of air resistance will equal to velocity to some power times some constant or F=kv^n. We have decided that the origin is where we first dropped the coffee filter and that the direction it is falling in is the positive direction. So if we did a Newton's Second Law equation, it would look like:
mg-kv^n=ma
a=g-(k/m)*v^n
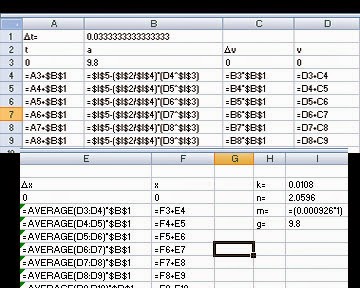
5. The next step is setting up a spreadsheet so we can decide what the values of k and n are. K and n describe the object's shape. We listed the Velocity and Force Resistance for each trial. We already know the velocity from the slope we got in LoggerPro and the Force Resistance at terminal velocity is simply the mass times the acceleration of gravity. We found from weighing 50 coffee filters that one coffee filter is 0.000926 +/- 0.000002 kg. From there we made a graph, did a power trend line, and obtained the following results:
From the given equation we now have values for k and n. k=0.0108 and n=2.0596. The values of k and n have an uncertainty of 5%.
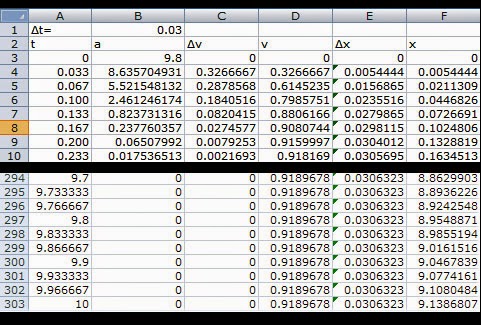
6. Now that we have an acceleration for for the coffee filters, we can plug in our equation into an Excel spreadsheet to see if our terminal velocity matches the one that we got from LoggerPro. Here are the visible equations we plugged in to show how we got our values:
7. In the cell for mass (I4) we can change how many times the mass is multiplied by to indicate the number of coffee filters we want to find the terminal velocity for.
For one coffee filter we have found from Excel that the terminal velocity is 0.9189678 m/s.
For two coffee filters we have found from Excel that the terminal velocity is 1.286648 m/s.
For three coffee filters we have found from Excel that the terminal velocity is 1.5665979 m/s.
For four coffee filters we have found from Excel that the terminal velocity is 1.8014375 m/s.
For five coffee filters we have found from Excel that the terminal velocity is 2.0075762 m/s.
8. We can now determine how close the terminal velocities we found were to the terminal velocities found in LoggerPro. The first trial with just one coffee filter, had terminal velocities that have a percentage difference of 5.10%. The second trial with two coffee filters, had terminal velocities that have a percentage difference of 4.48%. The third trial with three coffee filters, had terminal velocities that have a percentage difference of 5.40%. The fourth trial with four coffee filters, had terminal velocities that have a percentage difference of 4.10%.
The fifth trial with five coffee filters, had terminal velocities that have a percentage difference of 2.66%.
Conclusion:
Considering that k and n had a 5% error, our velocities turned out to be similar; however, 5% is still a significant difference in values. If we wanted to lower the percent difference of our values we would need more money to buy a better laptop, camera, and programs like LoggerPro and Excel, as well as more time to do the experiment.








No comments:
Post a Comment