PHYSICS LAB #2: Started on 2/25/15
Free Fall
Annemarie Branks
Professor Wolf
Objective: Prove that gravity will act up on a free falling object, with no external forces, at a downward acceleration of 9.81m/s^2.
Apparatus: An electromagnet is holding up a large piece of metal with has a metal ring around it that will come in contact with a wire. Once the magnet its turned off and the piece is allowed to drop freely, a spark will produce every 1/60th of a second and make a mark on the strip of paper parallel to it.
Description of Procedure and Results:
1. After the apparatus has made its marks on the strip of paper, tape down the strip on a flat surface to accurately measure the distance from your zero mark to each following mark. Record these distances. Note: The measurements should start from the end of the strip with marks that are closest together.
2. In attempt to find equations that represent the falling object's velocity and position (so that we can later derive a numerical value for acceleration) we must determine its distance with its corresponding time. We will also determine the mid-interval speed with its mid-interval time and produce a second equation. We are going to assume that the apparatus worked flawlessly and made a mark for every 1/60th of a second. From this information we can create a create a curved line and generate an equation.
3. The time that has passed for each mark will increase from the previous time by 1/60th of a second. In Excel we have generated a curved line and an equation to represent the relationship between distance and time. These were our results:
4. To calculate the increasing the Mid-Interval Speed we need to find its corresponding Mid-Interval Time which is simply 1/120th of a second in addition to the smaller time boundary of the interval.We determine the change in position by subtracting a distance from the following distance measured. Now Mid-Interval Speed can be determined by taking the change in position and dividing it by 1/60th of a second. With Mid-Interval Time versus Mid-Interval Speed, we can create a linear graph and equation to represent the velocity of the free falling object. These were our results:
5. To see how accurate this method of determining the acceleration of gravity is were going to derive the two equations.
Distance vs. Time
y = 479.44x^2+102.64x+0.2246
y' = 958.88x+102.64
y'' = 958.88 cm/s^2
The second derivative tells us the acceleration of the equation which is 958.88cm/s^2 or 9.59m/s^2.
Mid-Interval Speed vs. Time
y = 941.47x+105.92
y' = 941.47 cm/s^2
Since this is a Speed versus Time graph we only need the first derivative to determine the acceleration which is 941.47cm/s^2 or 9.41m/s^2.
Questions/Analysis:
1. Show that, for constant acceleration, the velocity in the middle of a time interval is the same as the average velocity for that time interval.
Since the velocity graph is supposed to be a straight line, the velocity in the middle of a time interval will be the average velocity for the interval. For example, if we chose the interval of t=0 to t=0.2 the average velocity should be the the velocity at t=0.1.
v(0) = 941.47(0)+105.92 = 105.92 cm/s
v(0.2) = 941.47(0.2)+105.92 = 294.214 cm/s
Average velocity for the time interval [0, 0.2]: (294.214+105.92)/2 = 200.067 cm/s
Is this velocity the same as the velocity in between times 0 and 0.2 seconds?
v(0.1) = 941.47(0.1)+105.92 = 200.067 cm/s
Therefore, the velocity in the middle of a time interval is the same as the average velocity for that time interval.
2. Describe how you can get the acceleration due to gravity from your velocity/time graph. Compare your results with the accepted value.
The derivative of velocity is equal to the acceleration.
y = 941.47x+105.92
y' = 941.47 cm/s^2
The accepted value for gravity is 9.81 m/s^2. In comparison, 9.41m/s^2 is not very accurate.
3. Describe how you can get the acceleration due to gravity from your position/time graph. Compare your results with the accepted value.
y = 479.44x^2+102.64x+0.2246
y' = 958.88x+102.64
y'' = 958.88 cm/s^2
Though the position equation produced a much more accurate acceleration due to gravity that the velocity equation, 9.59 m/s^ is still not very accurate when compared to the accepted value 9.81 m/s^2.
Assumptions made:
1. When the apparatus dropped the metal bar we assumed that it made no friction with the wire or the tape. Since the apparatus wasn't completely silent, there was friction which is why the Mid-interval speed vs. Time graph was not a perfect line. The friction would also explain why our determined acceleration of gravity was so much lower than the accepted value.
2. We also assumed that the apparatus worked perfectly in making marks exactly 1/60th of a second apart. If the assumption we made was wrong, this would also explain why the Mid-interval speed vs. Time graph was not a perfect line.
Uncertainty:
When measuring the marks on the tape we used a meter stick with centimeter and millimeter marks. We would then be uncertain of the hundredth place in our measurements when measuring in centimeters.
measurement (cm) = best estimate ± 0.01cm
Differences between expected and experimental values:
Absolute Difference = Experimental Value - Accepted Value
9.59m/s^2 - 9.81m/s^2 = -0.22
Relative Difference = (Experimental Value - Accepted Value)/Accepted Value * 100%
(9.59m/s^2 - 9.81m/s^2)/9.81m/s^2 * 100% = -2.24%
Errors and Uncertainty:
Using the all the lab groups results for acceleration we can determine the standard deviation from the mean. BY doing this we can statistically say how confident we are a range of values contains the actual value for acceleration due to gravity.
The equation for standard deviation is: (∑(x-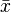
)^2 /N)^0.5
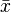)^2 /N)^0.5
We can create an empirical curve showing our confidence in our answer. For example "We are 68% confident that the actual acceleration of gravity is within one standard deviation of the mean. These values range from 935.91cm/s^2 to 976.16cm/s^2."
1. What pattern (if any) is there in the values of our values of g?
In the velocity graph, the points seem to be making a lesser than the average slope but then after three points it jumps up. I'd describe the pattern as slanted stairs.
2. How does our average value compare to the accepted value of g?
The average value differs from the accepted value by -24.96667cm/s^2
3. What pattern (if any) is there in the class' values of g?
There doesn't seem to be any pattern.
4. What might account with an difference between the average value of your measurements and those of the class? Which of these systematic errors? Which are random errors?
My partner and I made a systematic error when we were graphing our points. We repeated the last point twice which reduced our acceleration to 926.2cm/s^2 rather than 941.47cm/s^2. I hadn't realized our mistake until after we had shared our value with the class. I kept the value at 926.2cm/s^2 just so our standard deviation was the same as the rest of the class's.
In each trial, the apparatus dropped the metal bar differently which would explain why everyone had such different results.This would have changed the distribution of x, therefore this is a random error.
5. Write a paragraph summarizing the point of this part of the lab. What were the key ideas? What were you supposed to get out of it?
The purpose of collecting the class' data was meant to show that we could calculate an accurate acceleration of gravity; however, due to assumptions about the apparatus we ended up just proving that this was not the best method for finding the acceleration of gravity. The assumptions we made lead to most of the class's accelerations to be smaller than the accepted value.. By finding the mean of the class's accelerations and the standard deviation of the mean we can statistically say how confident we are in our range of values. Being able to put a statistic to our findings is what scientists should do when conducting their experiments and sharing them with the world. Without a statistic of certainty, you could being giving out false information.
No comments:
Post a Comment